Test No. VS15 Find maximum normal stress for two
configurations of a filleted bar.
Definition
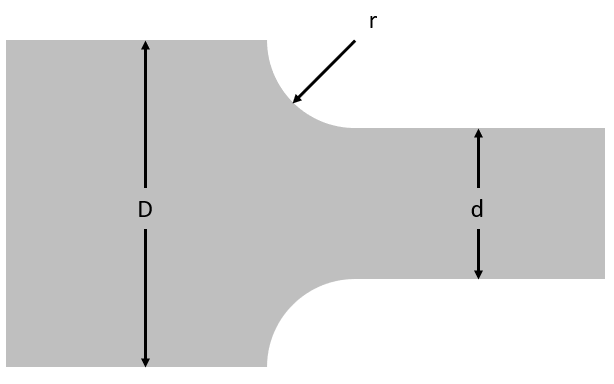
Figure 1.
Stress concentration is given by:
(1)
The following table outlines the two configurations of the loads on the bar.
Table 1.
| Dimension |
Configuration 1 |
Configuration 2 |
| D |
33 mm |
45 mm |
| d |
30 mm |
30 mm |
| r |
1.5 mm |
6 mm |
| t |
2 mm |
2 mm |
| D/d |
1.10 |
1.50 |
| r/d |
0.05 |
0.20 |
| Kt |
~2 |
~1.75 |
The material properties are:
- Properties
- Value
- Modulus of Elasticity
- 2.1e+11 Pa
- Poisson's Ratio
- 0.3
Results
Run
SimSolid analysis with 3 adaptive passes, Adapt to features and thin
solids on. The following table summarizes the stress results.
| |
Reference |
SimSolid |
% Difference |
| Max Prin. Stress - Configuration 1 |
2.00 |
2.04 |
2.00% |
| Max Prin. Stress - Configuration 2 |
1.80 |
1.84 |
2.22% |
